[Back] [Next] [Contents]
(Next is Part 3)

Hyperbolic Non-Euclidean World

Ch. 48 Conclusion for Part 2

  We have seen this way and that way. Now let us review a little.
We have felt;

Infinity depends on definition. Or, it might be better
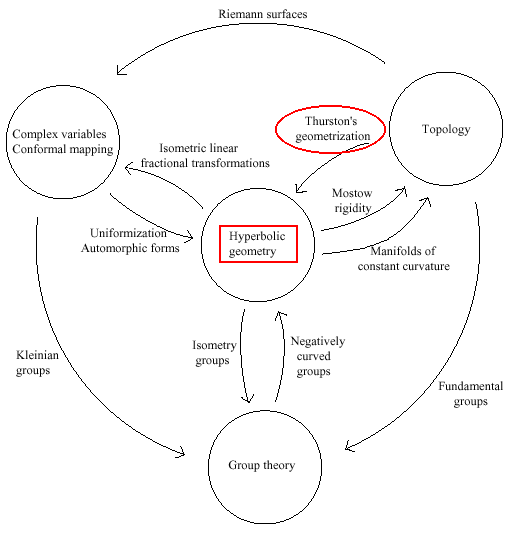
  to understand as an undefined term. In any case,
  it is imposible to make infinity as a mathematical
  subject.
Models simulate hyperbolic geometry perfectly, but not
  infinity itself.
Lobachevsky established hyperbolic geometry with
  postulate perfectly. But he relied on his own
  supposition about infinity itself.
  Also he judged the geometry stands up by insight
  without evidence.
Riemann said that we can go into any world by
  choosing a certain metric. But to give metric sounds
  intentional and not inevitable.
It is the most reasonable that we use projective
  geometry following Klein's Erlangen Programme. Be
  that as it may, we still feel something kile a
  conjuring trick.
We have not found a necessity to get into group
  theory.
According to pologists, almost everything around us
  has the hyperbolic geometry.


The following is histrical men and their works for the source of our story.

Euclid: He wrote that Euclid's Elements. (around BC 300)
        His logic has been so strong for more than 2000 years!

Pappus: He found the cross ratio is invariant. (around 320)

Mercator: He made the world map by using conformal mapping. (1538)

Napier: He invented the logarithm. (1614)

Newton and Leibniz: They invented differential and integral
        calculus. (around 1670)

Huygenes, Leibniz and Johann Bernoulli: They invented hyperbolic
        function. (1691)

Poncelet: He made projective geometry. (1822)

Lobachevsky, Bolyai and Gauss: They discovered hyperbolic geometry.
        (around 1826)

Gauss: He developed differential geometry. (since around 1827)

Laguerre: He expressed an angle with cross ratio. (1853)

Riemann: His idea was to construct a geometry by giving metric. (1854)

Beltrami: He gave us Pseudo-sphere. He pointed out that it has
        constant negative curvature. (1868)

Kline: He made a disc model (1871), and announced Erlangen Programme. (1872)

Poincare: He invented Upper Half Plane and disc models. (1882)

Thurston: He found that the Hyperbolic Non-Euclidean World is
        fundamental point of topology. (around 1975)


  Fig. 1 above is the closing entertainment for our mysterious sightseeing. It is made by Hayakawa, and named Hyperbolic Jungle Jim.

  Well, What's next? We will deal with so-called figure-8 knot that is closely related to hyperbolic geometry. And we are interested in Minkowski space, too.

  Fig. 2 below shows connections among hyperbolic geometry and other three fiels. It is copied from Hyperbolic Geometry by J.W. Cannon, W.J. Floyd, and W.R. Parry. Red marks that we added are our interest.


[Back] [Next(Part 3)] [Contents]

The End of Part 2 of Hyperbolic Non-Euclidean World