
Look at Fig. 1, which shows a tool that can be used to reproduce the Euclidean World as a model of the Elliptical Non-Euclidean World. The light blue hemisphere and the yellow disk are our model. Let's see how the tool works before we tell you why we are looking at these things.
Letfs draw parallel lines La and Lb on our desk in the Euclidean World. All
points P, Q, R, S, and any others on line Lb are projected to the center O of the hemisphere. The projection sweeps out as seen as the pink plane, which
intersects the hemisphere in a great circle t. Similarly, La makes another great circle u. End points at infinity on lines La and Lb are taken in the finite points a and b which are end points of the diameter of the hemisphere. As indicated by the red arrows, we get half-ellipses, t' and u', on the disk by orthogonal projection of the great circles, t and u. These half-ellipses, t' and u', have end points at point a' and b' at the edge of disk. We regard lines t, u, t' and u' as straight lines.
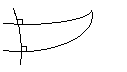
According to Georg Friedrich Bernhardat Riemann said, "A straight line in the Elliptical Non-Euclidean World is a great circle on a sphere model." If this is so, it would result that two straight lines intersect at two points, and would cause straight lines to be disqualified from straight lines. Then Riemann identified point a with point b. Isn't that a bit sly on his part?
This kind of technique is quite common today. He, also, constructed a geometry on the sphere that all antipodal (diametrically opposite) points are identified. That is elliptic geometry. Therefore our model is not the model of elliptic geometry. Nevertheless our model is very useful as far as we take infinity in our eyes. Moreover, our disk model is flat and easy to see. On the other hand the model of elliptic geometryis a sphere and you cannot see its entire surface at a time.
Since a great circle is regarded as a straight line, there are no parallel lines that are non-cross lines. Therefore we have to change our definitions of a straight line and parallel lines whenever we use the mechanism in Fig. 1 as a tool to enable us to go back and forth between the Euclidean World and the Elliptical Non-Euclidean World.
However, apart from theories, our tool works for drawing Euclidean plane with infinity. We can also see this situation in the Elliptical Non-Euclidean World. We conclude that we can draw the whole Euclidean world into a sheet of paper if we regard lines t and u and lines t' and u' as parallel lines, and points a and b and points a' and b' as points at infinity.
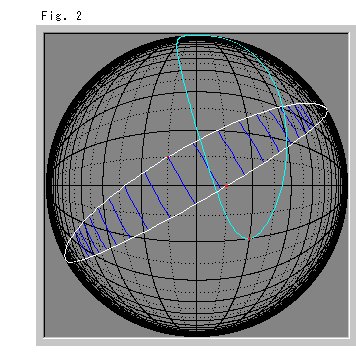
Now our yellow model disk in Fig. 1, when viewed face on, appears as the black disk in Fig. 2. It is a planar surface, but may be seen as the bottom of hemisphere. The black curved lines are to show spherical appearance. They are great circles with vertical and horizontal axis. The very edge is infinity in the Elliptical Non-Euclidean World. It seems that the disk's edge has no sense of angle or distance.
The two white lines are parallel, and therefore approach the same points at infinity. Just for your observation, the light blue curve is a parabola and the blue lines (which look like a ladder) are perpendicular to the parallel lines and touch them at regular intervals.
If we look at Fig. 2, in our model of the Elliptical Non-Euclidean World, we see that the parallel lines (white lines) meet at infinity (the outermost circle). They appear to form a loop, as does the parabola. Do they share anything in common at infinity?
Now let us tell you how we got this plane surface. To tell the truth, we had not aimed to see what is commonly called elliptical non-Euclidean geometry. It happened when we just tried to draw a picture at infinity since something infinite must be changed into something finite in order to be drawn on a sheet of paper.

So, we made the calculations shown in Fig. 3. Later on, we found that our formula is the same as that of elliptical geometry.
However, to make infinity finite for drawing, it is not necessary to use a hemisphere, or even the formula in Fig. 3. For instance, a circular cone or the formula, y = Arc tan x, will be sufficient. Of course, we could use a proper projective transformation.
So then, what is the Hyperbolic Non-Euclidean World? This is the question that we started to grapple with on our own journey toward the Hyperbolic Non-Euclidean World, and the result of our search is this homepage.