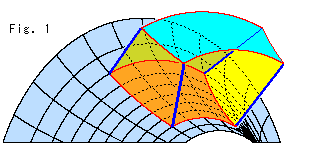
Fig. 1 shows a hut on the huge pseudosphere. The pillars look like they are leaning, but they are actually straight up (perpendicular) in parallel with the normals of the pseudosphere. The sky blue roof is hyperbolically flat (saddle-shaped). It would be a lot of fun if we live in this hut! All the furniture we bring in the hut is hyperbolic style. But the pull of gravity at every point has to be parallel with the normals. Otherwise, how can we live there?
If we are in space in a spaceship looking down at the Earth, when we come to Earth and get out of the spaceship, we lose all sense of the fact that the planet is a sphere. It is similar to the situation in the Hyperbolic Non-Euclidean World, even if we could stay in the hut. To fully grasp the nature of the Hyperbolic Non-Euclidean World, we need to have a considerably active imagination.
The hyperbolic plane is homeogeneous in every direction. Nevertheless, we carelessly look the pseudosphere as in deformed space due to its unbalanced shape.
We find an ingenious object. We can feel it with our hands and know what kind of world we are in at the time. It is a paper craft we are going to make.
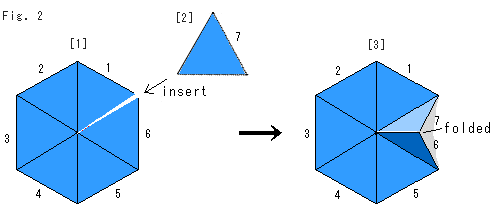

Look at Fig. 2. Take some cardboard or thick paper and cut it into equilateral triangles. You should make at least fifty triangles. Loosely tape six of them as shown [1] and add an extra triangle as shown in [2]. Then loosely tape them all and make a heptagon as shown in [3]. Because the taping is loose, we can connect seven triangles and make the heptagon though it no longer remains flat.
Fig. 3 is an actual heptagon. It is wave-shaped as shown.
Now add triangles around the heptagon in such a way that every vertex, as a common vertex, contains seven triangles. Be sure not to make a hole. We are making a surface. Just add one by one to the edge of surface we have made.

Fig. 4 is a photo after adding more triangles. This hyperbolic surface becomes floppier. We can even see the back sides of the triangles in the photo. No vertex is any more special than another. We can find no central point on the surface.
The paper craft is floppy, but regard it as a hyperbolic flat surface and crawl on the triangles as if you were a small insect. Compare this craft with pictures in chapter 4.
Kazushi Ahara developed a wonderful program called
Hyplane that utilizes (54-63-63 degree) isosceles triangles. (It makes the hole of a float, too.)
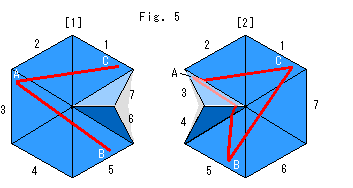
Look at Fig. 5. We draw a triangle ABC on the hyperbolic plane of paper craft. These pictures are an example drawn on the deformed heptagon of Fig. 3. But if we draw a large triangle, we should do it after making a paper craft that is large enough to allow us to do this easily.
[1] shows two red sides AB and AC drawn while keeping triangles 1 to 5 flat.
[2] shows the third red side BC drawn while keeping triangles 1, 2, and 5 to 7 flat.
We can ordinarily measure the angles and the length of sides of triangle ABC by deforming it like [1] or [2]. Even without measuring, we can see that the sum of the interior angles is less than ![]() , as shown.
, as shown.
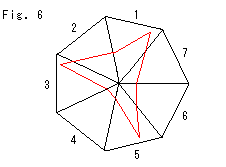
Look at Fig. 6. We pressed down the deformed heptagon (or compress it in the circumference direction) and got a flat regular heptagon. The red, closed, broken line is triangle ABC. It looks like a triangle in Poincare's disc, doesn't it? Of course, the angles and length are not the same as those of the heptagon in Fig. 5., but it helps us to get a feel for the hyperbolic plane qualitatively. This kind of diagram, however, is limited to a single heptagon.
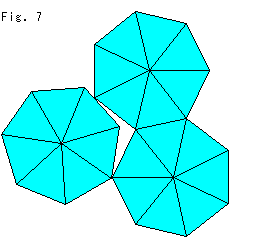
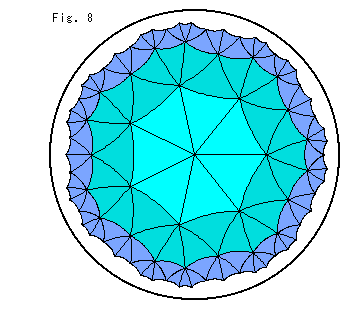
Fig. 7 shows that we can not help but have gaps for tilng flat heptagons. So we can not freely draw a figure on them. But, as we have learned it is possible on a hyperbolic plane.
Fig. 8 right is the tiling of regular triangles in Poincare's disc (cf. chapter 12). In this diagram, 111 regular triangles are drawn. The length of the sides and angles of every equilateral triangle is roughly:
side = 1.124 (hyperbolic length)
angle = 2![]() /7 = 0.8976 rad = 51.43
/7 = 0.8976 rad = 51.43![]() .
.
The triangles are separated by color like annuluses. The distance from the origin to the outer edge of the annuluses is roughly 1, 2, 3,,,.
Keeping this tiling in mind while envisioning ourselves crawling on the of the paper craft, we can get a feel for a hyperbolic plane.